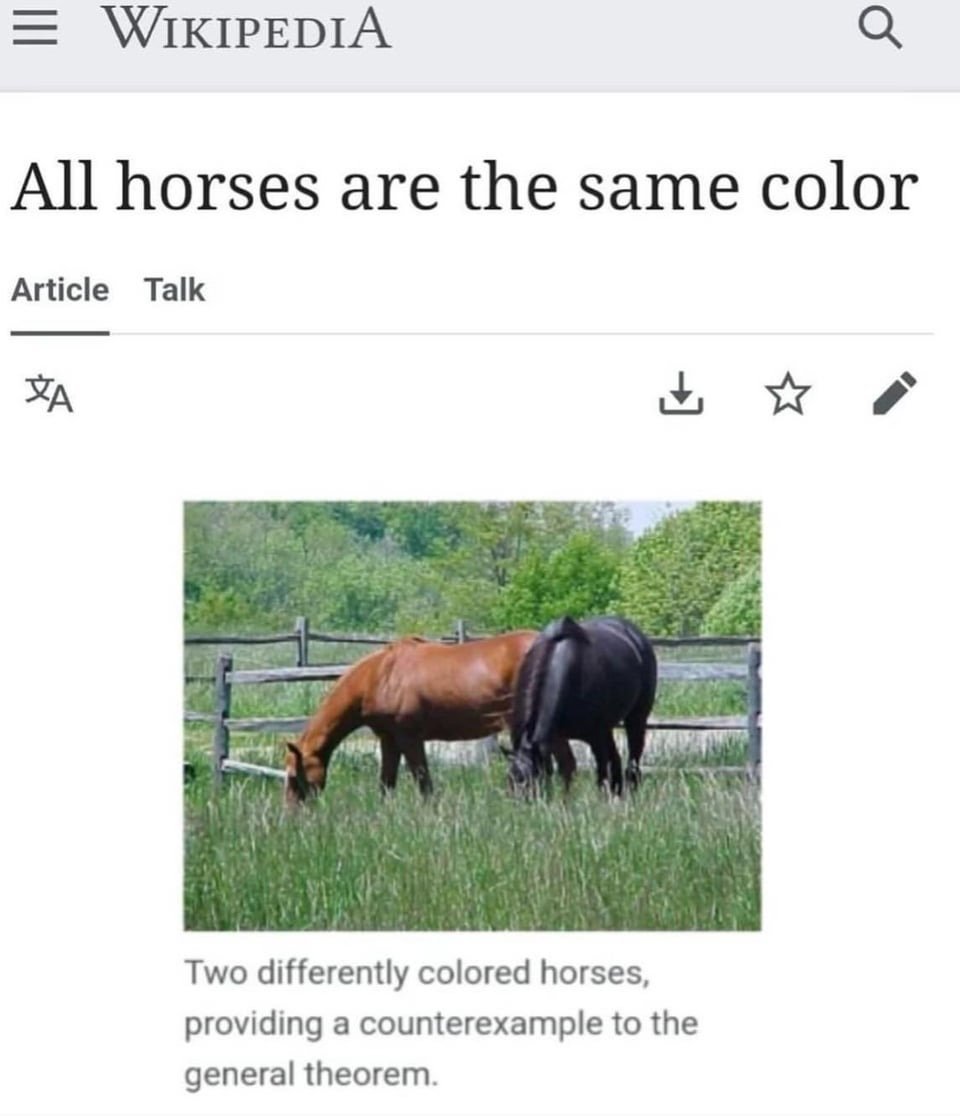
To be fair, until you can see both sides of each horse, that technically doesn’t disprove it
Lemmy Shitpost
Welcome to Lemmy Shitpost. Here you can shitpost to your hearts content.
Anything and everything goes. Memes, Jokes, Vents and Banter. Though we still have to comply with lemmy.world instance rules. So behave!
Rules:
1. Be Respectful
Refrain from using harmful language pertaining to a protected characteristic: e.g. race, gender, sexuality, disability or religion.
Refrain from being argumentative when responding or commenting to posts/replies. Personal attacks are not welcome here.
...
2. No Illegal Content
Content that violates the law. Any post/comment found to be in breach of common law will be removed and given to the authorities if required.
That means:
-No promoting violence/threats against any individuals
-No CSA content or Revenge Porn
-No sharing private/personal information (Doxxing)
...
3. No Spam
Posting the same post, no matter the intent is against the rules.
-If you have posted content, please refrain from re-posting said content within this community.
-Do not spam posts with intent to harass, annoy, bully, advertise, scam or harm this community.
-No posting Scams/Advertisements/Phishing Links/IP Grabbers
-No Bots, Bots will be banned from the community.
...
4. No Porn/Explicit
Content
-Do not post explicit content. Lemmy.World is not the instance for NSFW content.
-Do not post Gore or Shock Content.
...
5. No Enciting Harassment,
Brigading, Doxxing or Witch Hunts
-Do not Brigade other Communities
-No calls to action against other communities/users within Lemmy or outside of Lemmy.
-No Witch Hunts against users/communities.
-No content that harasses members within or outside of the community.
...
6. NSFW should be behind NSFW tags.
-Content that is NSFW should be behind NSFW tags.
-Content that might be distressing should be kept behind NSFW tags.
...
If you see content that is a breach of the rules, please flag and report the comment and a moderator will take action where they can.
Also check out:
Partnered Communities:
1.Memes
10.LinuxMemes (Linux themed memes)
Reach out to
All communities included on the sidebar are to be made in compliance with the instance rules. Striker
Doesn’t “color” (no ‘s’) imply they can’t be more than one? Or.. is this theorem further supported? Both of these horses are all the same color.
There are Value, Croma(or Saturation) and Hue, so maybe you're onto something here!
Actually a quite interesting article: https://en.wikipedia.org/wiki/All_horses_are_the_same_color
I did do this proof by induction back in the day, but now looking at the article I am clueless.
Do you mean you went through the proof and verified it, or falsified it?
As I understand it, it goes something like this:
...
You have a set of n horses.
Assume a set of n horses are the same color.
Now you also have a set of n+1 horses.
Set 1: (1, 2, 3, ... n)
Set 2: (2, 3, 4, ... n+1)
Referring back to the assumption, both sets have n horses in them, Set 2 is just incremented forward one, therefore, Set 2's horses are all one color, and Set 1's horses are all one color.
Finally, Set 1 and Set 2 always overlap, therefore that the color of all Set 1 and Set 2's horses are the same.
...
So, if you hold the 'all horses in a set of size n horses are the same color' assumption as an actually valid assertion, for the sake of argument...
This does logically hold for Set 1 and Set 2 ... but only in isolation, not compared to each other.
The problem is that the sets do not actually always overlap.
If n = 1, and n + 1 = 2, then:
Set 1 = ( 1 )
Set 2 = ( 2 )
No overlap.
Thus the attempted induction falls apart.
Set 1's horse 1 could be brown, Set 2's horse 2 could be ... fucking purple... each set contains only one distinct color, that part is true, but the final assertion that both sets always overlap is false, so when you increment to:
Set 1 = ( 1, 2 )
Set 2 = ( 2, 3 )
We now do not have necessarily have the same colored horse 2 in each set, Set 1's horse 1 and 2 would be brown, Set 2's horse 2 and 3 would be purple.
...
I may be getting this wrong in some way, it's been almost 20 years since I last did set theory / mathematical proof type coursework.
Yeah, so the following actually is valid: If all pairs of horses are the same color, all horses are the same color. Just starting the induction one step further.
Yep this is the exact issue. This problem comes up frequently in a first discrete math or formal mathematics course in universities, as an example of how subtle mistakes can arise in induction.
I took a peek and it is sort of dumb but logically "sound". Specifically the indictive step.
In the inductive step you assume the statement is true for some number n and use this statement to prove the statement n + 1 is true. If you can do that then you can prove the induction step.
So in this example the statement we assume is true is given n horses, all of them are the same color. To prove the statement for n + 1 horses we look at the n + 1 horses. Then we exclude the last horse. By excluding the last horse we have a set of n horses. By the induction statement this set of horses must all be the same color. So now we've proven the first n horses are the same color.
Next we can exclude the first horse. This also gives us a set of n horses. By the induction statement all these horses must also be the same color. Therefore all n + 1 horses must be the same color.
This sounds really dumb but the proof works in the induction step.
The logical issue is that the base case is wrong which is necessary for a complete proof by induction.
Also related: https://en.wikipedia.org/wiki/Raven_paradox
A much more interesting question, if you ask me.
It was stated as a lemma, which in particular allowed the author to "prove" that Alexander the Great did not exist, and he had an infinite number of limbs.[4]
Talk about burying the lede! 😄
From that link:
Assume that n horses always are the same color.
... I mean.... yes, the logic follows... if you... make and hold that assumption... which is ostensibly what you are trying to prove.
This is otherwise known as circular reasoning.
Apparently this arose basically as a joke, a way of illustrating that you actually have to prove the induction is valid every step of the way, instead of just asserting it.
EDIT: As others have pointed out, the fallacy here isn't the circular reasoning fallacy.
It is however a logically/mathematically invalid attempt at proving induction.
It doesn't logically/mathematically fail because of the assumption of horse color, that's just taken as part of the givens before the argument really begins.
The problem arises elsewhere, I tried to work through exactly where in another comment.
No, that's what induction is. You prove the base case (e.g. n=1) and then prove that the (n+1) case follows from the (n) case. You may then conclude the result holds for all n, since we proved it holds for 1, which means it holds for 2, which means it holds for 3, and so on.
Exactly, the assumption (known as the inductive hypothesis) is completely fine by itself and doesn't represent circular reasoning. The issue in the "proof" actually arises from the logic coming after this, in which they assume that they can form two different overlapping sets by removing a different horse from the total set of horses, which fails if n=1 (as then they each have a single, distinct horse).
You are correct that in the mathematical sense, this is not circular reasoning, it is induction.
The problem is that this is an example of a failed, invalid proof of induction.
I investigated it a bit further and tried to work through the actual point at which the proof fails in another comment.
It’s not circular reasoning, it’s a step of mathematical induction. First you show that something is true for a set of 1, then you show that if it’s true for a set of n it is also true for a set of n+1.
As with Kogasa, you're right that this is not circular reasoning, it is induction.
I judged it a bit too quickly.
However, it isn't a valid proof of induction.
I tried to work through exactly where and how it fails in another comment.
So... it is still fallacious reasoning of some kind, but yes, not the circular reasoning fallacy.
Actually this just refers to the color of their fur. I'd say that a blonde white man and a white read head are the same color, just their hair has a different color.
(I'm not totally serious and will not die on this hill)
Oh, I'm gonna make sure you die on that hill!
First, by building you a lovely house on that hill and a nearby Denny's...
Sounds nice, go on!
(I'm gullible and will fall into any trap I come across)
I'm not even convinced that horses are real.
They’re what the government uses to spy on the Amish.
Why can't they just use birds like for everyone else?
I'm not convinced that anything outside my own thoughts are real. In fact are my thoughts real?
You're not real. I am not real. Nothing is real.
True. They look too similar to giraffes
Why is there a picture of an empty field?
I assume John Cena is standing there and we just can't see him
This is what arguing with people about politics feels like.
In some cultures this might be the same color.
They are both horse colored, obviously.
Their hair is the same color. What color is the skin?
Horse blindness is a made up illness designed to gaslight us into believing that horses exist.
That theory assumes that color is real. Color is just some photons smacking into your retina and then the brain deciding what that even means.
Well, sorta. Different materials bounce different wavelengths of light that our eyes catch and send to the brain to piece together and interpret color from. There is a degree of responsibility for what we see on the object the light bounces off of in the form of what wavelengths they absorb or reflect.
Aha, but that assumes light is real and materials are real and the universe is real!
But the reasoning given doesnt apply exclusively to horses. Suppose we follow the same chain that gets us "all horses are the same color", but replace "horses" with "colors", we would end up with the statement that all colors are the same color. Thus, this is not a counterexample, because black and brown are the same color.
On the inside?
...what?
Horse hair colors vary.
If you shave a horse, any breeds with dark black or blue-ish grey hair will give you a variety of that pallet, getting dark enough to be stopped and frisked in larger American cities. Sometime blueish-black skin and hair pigmentation matches as well.
Most other breeds will give you a pink color range.
https://artpictures.club/autumn-2023.html
Source: was given a saddle for Christmas once.
It's not actually claiming that all horses are the same color, it's an example of a flawed induction argument
If you read the Wikipedia article (or other articles) about "all horses are the same color", it is really just about an inductive fallacy that can occur.
Mammals only make brown, but we do a lot with it.
Maybe author of the sentence was looking at horses in space and wikipedia is completly wrong.
This is great. I love stuff like this.